
In this post, I discuss to what extent the concept of invariance can connect historiography, sciences, and futures studies. I argue that we can find invariances concerning human activities, especially in the case of science. I argue that (a) science, historiography, and futures studies require knowledge of invariances, and (b) knowledge of invariances enable us to locate ourselves in the causal processes between the past and the future.
Invariance refers to the property of remaining unchanged or constant while some other conditions change. In this post, we focus on the invariance of generalizations. A generalization G is invariant with respect to changes C if and only if G holds when C takes place. To give some simple examples, Newton’s laws describe how a spacecraft behaves on earth; and the laws hold when the spacecraft is on the moon. The laws are invariant with respect to the change in location. On the other hand, Newton’s laws do not describe how an object behaves when its approaches the speed of light. The laws are not invariant with respect to such changes in the speed of an object. They do not hold in such conditions. (Note that there are much more abstract invariances: If Newton’s laws are valid in one inertial frame of reference, then they are valid in any frame of reference in uniform motion with respect to that frame of reference).
The generalization if a barometer falls, a storm appears is invariant in a wide range of weather systems. However, the generation is notorious for its deceptive causal association. That a barometer falls does not cause a storm to appear. Its range of invariance is irrelevant from the causal point of view. If we were to manipulate the barometer and make its reading fall, a storm would not appear. The generalization is not invariant under interventions and therefore does not describe a causal relationship. (See Woodward 2003.) I come back to this later.
Following the consideration above, we can make a distinction between (a) invariance with respect to background conditions and (b) invariance with respect to interventions. A generalization can be invariant in many background conditions while not being invariant with respect to interventions (i.e. causal). The relationship between barometers and storms is invariant in this sense. On the other hand, a generalization can be invariant with respect to an intervention while holding only in specific circumstances. A shy person may sing in his home when asked but refuse to sing elsewhere. The person will sing in his home when asked (i.e. a generalization holds between asking and signing) but the generalization fails to hold in other circumstances. Finally, there are generalizations that hold in a wide variety of background conditions and are invariant with respect to interventions, such as Newton’s laws.
Invariant generalizations are central in science because they enable us to (i) organize our knowledge in an economical way and (ii) separate causal generalizations from non-causal ones. Given that we improve the invariance of scientific generalizations, the scope (“the range of applicability”) of those generations increases. Moreover, the ability to separate causal generalizations from non-causal ones provides us with insight on how to effectively manipulate phenomena.
So far so good. Invariant generalizations are important in science. But what about the human and social world? Invariant generalizations (i.e. generalizations that are invariant to some degree) seem like a promising candidate to connect (a) natural and (b) human and social sciences (HSS). We do not require strict laws that are hardly ever found in HSS but generalizations that remain true in some relevant set of different conditions.
The problem is that the generalizations in HSS are messy and conceptually unclear. Consider the democratic peace hypothesis: Democracies do not fight wars with each other. As Goertz has pointed out, it has at least three problematic concepts: war, state, and democracy. What counts as a democracy, state, or war is critical in the validity of the generalization. (2012, 100.) In many relevant cases, it is difficult to tell whether the case belongs to the scope of the generalization. If we are not able to identify unambiguously the cases that belong to the scope of the generalization, then it is difficult to gather evidence against the generalization. If the generalization fails to hold in some case, one can always question whether the case involved genuine democracies, states, or war. Difficulties in identifying the cases that belong to the scope of the generalization also means that the use of the generalization in the estimating of futures is equally difficult. The only thing we can predict on the basis of the generalization is that there will not be genuine war between genuine democratic states. Such prediction is silent about real-world messiness concerning political systems and conflicts. For example, if a proxy war does not count as a genuine war, the generalization is silent about an important issue, the possibility of certain proxy wars in the future.
In essence, the problem has two dimensions. First one is epistemic. Because HSS cannot conduct systematic experimentation, it is impossible to establish what types of political systems avoid wars and what kinds of wars they avoid. For example, Goertz points out that “Finland and the United Kingdom in World War II is a case of two democracies fighting. However, this conflict is very minor in the context of World War II, which itself supports the democratic peace hypothesis” (2012, 100). However, we cannot make experiments and change the historical UK or Finland to non-democracies and see whether the conflict would have been more severe in those conditions.
The second problem is metaphysical. Human societies, cultures, and activities change. This is the essential lesson from historiography. For example, political systems and the nature of armed conflicts change. Because of this, it is rather difficult to find strict and clear generalization in HSS. If we look at the history and attempt to find regularities (for example, between democracies and wars) we always need to ask in what sense two historical entities (e.g. political systems) or historical events/processes (e.g. war) are similar enough to be subsumed under a generalization. There are always differences between historical entities and processes and these differences seem to make the generalizations in HSS messy and unclear.
When pushed to the extreme, this view ends up in historicism:
“Historicism. There are no permanent invariant properties. All objects and properties are temporal and subject to variation”. (Kuukkanen 2016, 5.)
Historicism implies that general knowledge concerning the development of human societies, cultures, and activities cannot be achieved. There are no useful generalizations concerning these phenomena. In terms of invariance, historicism states that generalizations concerning HSS phenomena are not invariant with respect to temporal changes.
In the history and philosophy of science, this problem is also called Heraclitianism: “Scientific concepts, experimental methods and standards, and even the notion of science itself shift from one historical moment to another” (Bolinska and Martin 2020, 50). Heraclitianism implies that there are no generalizations concerning scientific activities that remain invariant with respect to temporal changes.
This issue of temporal variance requires serious attention and explanation if we want to understand the relationship between natural world and human beings and the relationship between HHS and natural sciences. The temporal variance is also central issue in the foundations of futures studies. The question is how we can reconcile the idea of temporal variance with the necessity of generalizations in the estimating of futures.
Osmo Kuusi has analyzed the philosophical grounds of futures studies and has interesting things to say about the concept of invariance in futures studies. In his General Theory of Consistency, Kuusi distinguishes between (a) not-learning and (b) learning beings:
“A not-learning being with invariant criteria of sameness of its behavioral language always behaves (reacts) from the point of view of those criteria in the same way in the same situations.” (2019, 26). To continue our example above, consider a spacecraft that is send to the space. This is a not-learning being that reacts to its surrounding conditions in accordance with the fundamental laws of nature. No matter what the surrounding conditions, the behavior of the spacecraft remains invariant from the point of view of the fundamental laws. In other words, the fundamental laws are the real invariances of the object; they define the behavior of the object in all possible situations. However, it is important to notice that the known laws of nature we have found are not, exactly, the fundamental laws. For example, Newton’s laws are invariant within a certain range of conditions and describe the behavior of the spacecraft in those conditions. Also, the laws enable us to predict and manipulate the behavior of the spacecraft within their range of invariance. Yet, Newton’s laws break down if the spacecraft’s speed approaches the speed of light.
It is important to note the distinction between real and known invariances. In the case of not-learning beings, we assume that there are real invariances that our known generalizations approximate. While we can improve our stock of generalizations with respect to their invariance (for example, Galileo-Newton-Einstein was a chain of such improvements), the real invariances of the not-learning beings do not change (by definition). When we develop new generalizations that describe the behavior of not-learning beings, this is not due to the fact that these objects changed their behavior. Rather, new generalizations are formulated in order to have more invariant descriptions of their behavior. There is nothing wrong with the old generalizations except that their invariance is limited, and we need a better approximation of the real invariances. On the other hand, a failure of presumably invariant generalization to describe the behavior of a not-learning being in the known conditions of invariance falsifies the generalization. This is an important difference between our approach to learning and not-learning beings. Let’s see why.
“A genuine learning being (actor) is able to change its criteria of sameness in a way that falsifies the predictions based on its past behavioral patterns. [–] Also the behavior of an actor is determined by its behavioral language. Besides its behavioral language, the actor has mental or sense-making language(s) that it uses to control its own behavior; in prediction of the behavior of not-learning beings; and in the anticipation of the behavior of other actors and in the communication with them.” (Kuusi 2019, 26-27.)
For example, a scientist who attempts to send the spacecraft to the Moon is a genuine learning being. The scientist attempts to predict the behavior of the spacecraft during the journey. If we attempt to predict how she will model the journey, we will not have a guaranteed success. For if she is unable to model the journey of the spacecraft, she will learn that the existing modeling-practice does not work and she will attempt another approach.
In the case of learning beings, the prediction of their behavior is difficult or even impossible in many cases: “[In] the case of a learning being, a wrong prediction does not necessarily tell, that a researcher was earlier wrong concerning the criteria of sameness of the being. The learning being may have changed her criteria of sameness. The basic criterion for the change is an experience of contradiction or inconsistency: an inconsistency between results of an action and the interests of the being or an inconsistency between anticipated and actual results of the action.” (Kuusi 1999, 18.)
The behavior of a learning being is not chaotic or incomprehensible in terms of generalizations. The problem is that the generalizations that describe the behavior of a learning being are not invariant with respect to learning. Nevertheless, they are invariant to some extent:
“Because the learning can change these invariances – pattens of behaviour, traditions, expressed preferences, images of the future – I call them transient invariances in comparison with the (permanent) invariances of not-Iearning beings (Kuusi 1999, 19).
The existence of transient invariances provides stability and predictability to human activities, although in limited contexts. In this way, they can potentially work as basic units of analysis of human activities.
Kuusi formulated his framework with the explicit intention to provide philosophical grounding for futures studies. In what follows, I will discuss how the notions of permanent and transient invariance serve this role and I will argue that the notion the two notions of invariance are especially useful in connecting historiography and futures studies despite historicism and Heraclitianism: The two notion enable us to understand how historical understanding is possible. We will see that different types of invariances enable us to connect historical particularism with general considerations and to locate ourselves between the past and the future.
Obviously, permanent invariances of not-learning beings can be used to estimate possible futures and explain the past. For example, evidence for the Little Ice Age can be found through techniques for assessing historical temperatures, such as tree rings. These techniques are based on known invariances in nature such as those that determine the growth of a tree. In the same way, known invariances can be used to estimate that carbon dioxide emissions will warm the Earth and to estimate how the living conditions will change due to the warming. The permanent invariances enable us to estimate (i) the contexts where future action will take place and (ii) the consequences of our actions (e.g. carbon emissions).
The real issue in futures studies is how to estimate the future of human actions and societies. Even when we know the transient invariances that govern human activities, we may fail to estimate how people act. Transient invariances change as people learn. The key is to understand the learning processes and the space of possibilities that is allowed by the processes. Kuusi writes:
“But how do we falsify the assumptions concerning the criteria of sameness of learning beings or how to predict the changes of their criteria of sameness? [–] The evidence has to concern the learning processes of the learning being. Learning processes are often non-deterministic. There are often many choices, within the capability limits [–] of learning being.” (1999, 33.)
Let’s focus on scientific activities (after all, the name of this blog is Futures of Science). In science, the learning processes appear to be somewhat transparent, at least on the surface. Consider the following case. In the 1900th century, the astronomical tables of the orbit of Uranus were published, making predictions concerning the orbit on the basis of Newton’s laws. It turned out that the actual orbit deviated from the one that was predicted. In this case, the scientists, as learning beings, realized that the attempt to model the orbit would not succeed on the basis of existing knowledge and Newton’s laws. They would need to change the way in which they build the model. There were roughly two options: Either Newton’s laws are to be rejected or the existing knowledge concerning the solar system updated. Scientists made the hypothesis that there exists an unknown planet that have an effect on the orbit of Uranus. The unknown planet, Neptune, was discovered in 1846.
On the surface, this case is simple. The learning process that changes how models are built includes either abandonment of known theories or an attempt to improve the background knowledge. Surely, we cannot know which strategy will work in any given case, but the overall structure of the learning process remains comprehensible in the case of science. This surface image is apparent in science policy discussions, where research projects are framed in terms of clear strategies and possible outcomes of various situations. However, in order to understand the deeper problem in our knowledge of futures of science and transient invariances, we need to look below the surface.
Notice, first, that the case above can be explained by many theories of the philosophy of science. (In fact, the case is so canonical that any plausible theory in the philosophy of science probably needs to be able to give an account of it.) In Kuhn’s terms, the episode consists of normal science doing what it does best: Scientists use an established framework (e.g. Newtonian mechanics) and attempt to solve a problem (e.g. predict the orbit) using established techniques (e.g. calculation, telescope observation) within a given ontology (for example, the existence of one more planet was rather minor ontological commitment in 1900th century[1]). In Lakatos’s terms, scientists protected the hard core (Newtonian mechanics) of their research program by altering the protective belt (what planets exists) and, in this way, the scientists were able to keep their research program progressive.
Thus far, philosophical theories appear to make a simple point in more sophisticated terms. But this is not the whole story. Consider another case, the precession of the perihelion of the orbit of Mercury. All planets, including Mercury, travel in elliptical orbits. The ellipse is not fixed. It gradually rotates, so that Mercury’s point of closest approach to the Sun – called the perihelion of the orbit – drifts, or precesses. In the mid-1900th century, a problem appeared: The precession of the perihelion of the orbit of Mercury did not match the calculations from Newton’s theory. What to do? One could change the estimated mass of Venus to account for the difference. However, this would affect the movement of the Earth in a way that had not been observed. Then the scientists considered the Neptune-trick: Perhaps there exists a planet (Vulcan) even closer to Sun that causes the difference. However, no planet was found, and the mystery remained. Only after Einstein had formulated the Theory of General Relativity, the problem could be solved. In this case, the Newtonian physics needed to be replaced by Relativistic physics in order to solve the anomaly.
No philosophical theory could have predicted the formulation of the Theory of General Relativity. However, the theories make fundamental theoretical change in physics understandable by placing it in a theoretical scheme. For example, Kuhn underlined the existence of scientific revolutions in which fundamental theoretical changes occur along with fundamental changes in scientific activities (in methods, norms, values, techniques etc.). The theory enables us to see that the simplistic piecemeal model of scientific improvement through minor theoretical adjustments or new factual knowledge is not the whole story. Surely, there was a theoretical change and improvement – a big one – but it happened over a half century after the problem of precession was recognized and it happened independently of that particular problem.[2] The philosophical theories of science can therefore enable us to understand the nature, possibilities and obstacles in the learning processes in the scientific activities. They describe the invariances that govern the variance produced by learning processes.
I have discussed in an earlier post how theoretical frameworks (like the ones produced by philosophy of science) can be understood as descriptions of possible structures of the future. In terms of invariances, we can take these theories as providing invariant generalizations concerning the nature, prospects, and obstacles of learning in a given field. For example, the Kuhnian theory suggests that the future will consists of periods of (I) normal science when (a) problems are solved in a piecemeal manner in the rather strict limits of a paradigm and (b) some stubborn problems (anomalies) are left open (e.g. the precession of the perihelion) and (II) revolutions when the space of possibilities for problem solving and rearrangement of scientific practices opens radically in the face of anomalies.
The considerations above suggest that there exists a hierarchy of invariances concerning human activities. On the one hand, there are low-level invariances that concern activities until a learning process begins, such as the one that describes a particular attempt to model a phenomenon such as the precession or the orbit of Uranus. In addition, there are mid-range invariances that concern the use of certain resources to solve a problem in a learning process, such as the paradigm-driven normal science project. Finally, there are structural invariances that concern the prospects and limits of any learning process in a given field, such as Kuhnian anomaly-crisis-revolution structure where anomalies are generated by strictly limited learning resources of a normal science up to a point when the available resources are abandoned and replaced by a wide open space of possibilities.
The existence of different levels of invariance suggests that the concept of learning can serve to connect different historical steps without committing to some problematic notions of growth of scientific knowledge. We do not need to think that science is a linearly cumulative practice (see a previous post on the problems of such view) in order to tie together its different phases. Neither do we have to accept a “capsule view” of history where different contexts are studied in order to understand the sheer singularity of times and places and nothing more – a view that, ultimately, obscures us from our historical underpinnings. We can understand the development of science in terms of learning processes, i.e. by studying the structure, prospects, and limitations of learning in different historical situations. Moreover, we have seen that philosophical theories might be a good starting point for the analysis of the structural invariances that govern the development.
However, the problem of historicism is still not completely solved. If it is a fact of history that science changes and follow patterns that are invariant only in very limited contexts, the prospects of using invariances in the estimating the future of science remain rather grim. Even if we can find out some structural invariances that govern the space of possibilities of any learning process, the context-dependence of science, presumed by historicism, makes the search of invariances useless. But is such radical context-dependence a historical fact or a historiographical insight?
Consider the following case: In the paper “Inspiration in the Harness of Daily Labor”, Richard Bellon (2011) describes the process that led to the acceptance of Darwin’s evolutionary ideas in the British scientific community. Bellon claims that the publication of On the Origin of the Species in 1859 was not a decisive event in this process. On the contrary, the book was judged to be speculative in character and against the scientific and moral standards of the community. Only after publishing a study on orchid fertilization, in which he applied his evolutionary ideas, was Darwin’s framework meet with approval by the community.
Bellon is providing an answer to the question:
Why were Darwin’s ideas accepted by the scientific community rather than not?
Bellon gives the following answer:
“The Origin was packed with evidence, but it communicated few entirely original scientific observations, and this allowed its critics to dismiss it as vainglorious speculation untethered from the manly discipline of original discovery. […] If Darwin had not tied the theory of the Origin to productive, technical, and specialized research — in the event, his floral biology, but it could have been any number of things — the ultimate reception and received meaning of the Origin would have been significantly different. […] Science’s social, political, and religious respectability depended on the governance of imagination by consistently patient and humble behavior and […] Darwin’s adversaries frequently used this ideology to bludgeon the Origin. Ultimately, Darwin vanquished his foes by reversing the weapon and claiming the mantle of heroic conduct for himself and his theory.” (395-396.)
It may seem that the explanation is a rather straightforward one, at least for a philosopher of science. Darwin first introduced a theory in the Origins, but since the evidence for the theory consisted of “old evidence” rather than of novel discoveries that the theory entails and since novel discoveries are important in the confirmation of a theory, Darwin’s theory was not well confirmed and thus not accepted. What is more, his theory had not then been shown to produce what Lakatos would have called a progressive research program and, because it seemed like an improbable candidate to produce one, it was not accepted. Once Darwin published the Orchids, his evolutionary framework proved to be a fruitful research program and to produce novel discoveries and was accepted. The importance of novel discoveries and fruitful research programs in scientific activities are well known invariant properties of science, at least for philosophers. The explanation of the acceptance of the evolutionary framework can be formulated as follows:
(E1) Had Darwin not shown in Orchids that his framework was successful and progressive (i.e. fruitful) in empirical research, the evolutionary framework would not have been accepted.
The explanation is based on transient invariant generalizations: “Scientific framework is accepted when it produces new findings and is fruitful in empirical research.” This generalization has not always been the case; for example, mathematical science was connected to empirical sciences in the process of scientific revolution (as mentioned in the previous post). However, the generalization does not seem to be restricted to any local context of science but is able to describe a wide range of scientific practices.
Yet, there is a complication in the issue. Bellon also points out that before the Orchids was published, Darwin’s work was dismissed on moral standards. Darwin did not seem to work on a patient, humble and honest manner and, therefore, was not taken seriously. Only after the Orchids, was Darwin accepted as a morally righteous scientist, and therefore the fulfillment of Victorian moral standards was an important factor in the acceptance of his theory. As Bellon points out, the requirements for novel discoveries and progressive research program (i.e. the requirement of fruitfulness) were an embodiment of the Victorian value system and moral standards.
This explanation for the acceptance of Darwin’s theory can be framed as follows:
(E2) Had Darwin not lived in accordance with the values of Victorian society, his evolutionary framework would not have been accepted.
This explanation in based on generalization “Theory is accepted only if it is produced in accordance with the vales of Victorian society”, and the invariance of the generalization is obviously limited. Prima facie, this case seems to provide evidence for historicism: A local and limited context, Victorian society and value system, governed scientific development.
However, the case does not support historicism. There is an asymmetry between E1 and E2. It can be seen if we consider the following generalizations:
(I) Theory T is accepted if it is fruitful, and
(II) Theory T is accepted if it is formulated by a scientist living in accordance with the moral values of the society.
There have been theories that have been accepted due to their fruitfulness despite the moral condemnation of the scientist. The case of Galileo Galilei is probably the best known. E1 and the associated generalization (I) seem to deserve their place in our explanatory resources. Producing fruitful results seems to be much more invariantly connected with the acceptance of a theory than the moral virtues of scientists. In Darwin’s case, the close connection between fruitful scientific practice and moral virtues was rather coincidental.
Moreover, there are important changes in background conditions that would have made E2 false, i.e. there are important changes with respect to which E2 is not invariant. For example, nothing indicates that had Darwin done something morally wrong that was not related to his scientific work, his theory would not have been accepted. In other words, it seems possible that had Darwin done something morally wrong (perhaps some minor misbehavior) and produced his theory, his theory would still have been accepted. Moreover, it seems possible that had Darwin lived in another society, his theory would still have been accepted even if he did not follow the Victorian value system.
However, it is difficult to figure out any change in background conditions that would have made E1 false. What should we add to the claim “Had Darwin not proved his theory fruitful and progressive, it would still have been accepted” to make that claim true? Of course, we can say that had Darwin lived in a society that considered fruitfulness as a reason to reject a theory, his theory would not have been accepted. However, it seems difficult to tell what kind of change this would have required. The claim seems to have the form “Z would not have been the cause of X if Z occurred in the context where Z does not cause X,” and thus lacks informative content. This mean that E1 is invariant with respect to wider range of important and meaningful background conditions than E2.
One way to look at the difference between E1 and E2 is that one can abandon Victorian moral standards and still perform fruitful scientific work. However, it is not possible to abandon the standard of fruitfulness and still live in accordance with Victorian moral standards, as the standard of fruitfulness is an embodiment of the moral standards. The antecedent of E2 is never true when the antecedent of E1 is not true. However, the antecedent of E1 can be true even if the antecedent of E2 is not true. E1 can remain true in conditions where E2 is false, but E2 is never true in conditions where E1 is not. The range of invariance is much greater for E1 than E2.
The case illustrates the point that we can discover invariances in historical phenomena even if the phenomena appear context-sensitive. However, we should still appreciate the methodological lesson implied by historicism: Knowledge of scientific dynamics cannot be achieved except by looking at concrete historical cases (see detailed argumentation in Virmajoki 2021). We have to search for similarities and differences between cases. The cases of Galileo and Darwin speak for the relationship between fruitfulness and scientific acceptance; the case of Lysenkoism seem to indicate that the moral or political values of scientists sometimes do, in fact, matter more than the fruitfulness of a theory. Science does change and its dynamics are complex. Crude universalism that attempts to capture science once and for all by a limited set of factors is therefore an unlikely candidate to be the correct approach to the study of science. We need to look at historical cases in detail in order to understand those dynamics. This leads us to the final issue of the post.
Not every invariant generalization describes causal relationships. While we can use non-causal invariances (like the relationship between barometer readings and storms) to estimate the future, those relationships are inferior to causal ones because they do not enable us to manipulate the world.
For example, consider the following interpretation of Darwin’s case:
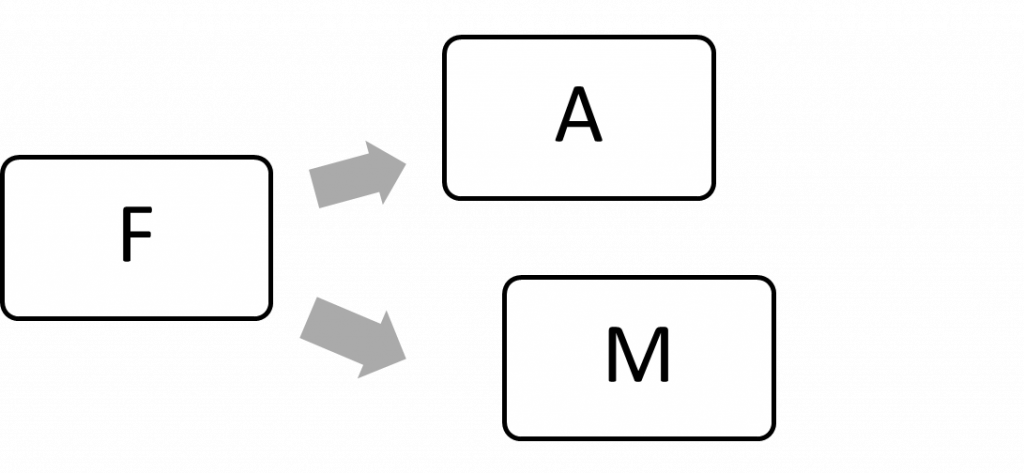
F is the (known) fruitfulness of a theory, A is theory-acceptance, and M is moral approval. First, Darwin’s theory was not known to be fruitful, and Darwin was not morally approved, and theory was not accepted. Then the theory was proven to be fruitful, and Darwin was morally approved, and the theory was accepted. Now, it seems that it is true that “Had Darwin not been morally approved, his theory would not have been accepted” is true. Yet, this counterfactual does not establish a causal relationship between moral approval and acceptance. It is not invariant under an intervention on A.
“The intuitive idea is that an intervention on X with respect to Y changes the value of X in such a way that if any change occurs in Y, it occurs only as a result of the change in the value of X and not from some other source.” (Woodward 2003, 14.)
The only way to change A in order to change M is to change F. But if F is changed, the change in A is not due to the change in M but F. This way of changing M does not count as an intervention (with respect to A) and therefore the relationship between M and A is not invariant under an intervention (and therefore not causal).
The point here is that even if we know the invariant (with respect to background conditions) relationships between F, A, and M, their causal relationships still require further analysis. Moreover, in order to understand how to achieve certain goals, we need to understand the causal relationships. For example, we could be misled to think that strengthening the moral status of scientists working in a project is an effective way of improving the status of their results in science by looking at the history of science. However, such improvement would be futile if it did not concern the epistemic improvement of their work. This also has a normative implication: It is important that we keep on track which attributes of scientific projects are rewarded. Virtue signaling in the absence of worthy epistemic achievements should not be rewarded.
We also need to understand that the net effect of any factor on the outcome of a scientific process depends on other factors as well. Even if there are invariant relationships that govern scientific processes, different combinations of such invariant relationships in a particular historical situation might be unique (see Virmajoki 2021). Therefore, it is important to study historical situations in detail. Even if we were confident that certain invariances govern the development of science, the interplay of the invariances depends on the case at hand. For example, both moral and political values and fruitfulness probably have an effect on scientific processes. In different contexts, their effect can differ. In the case of Darwin, the interplay was favorable to the fruitfulness and ultimately scientific progress, and in the case of Lysenkoism, the interplay led to a politically corrupt and degenerating science.
Moreover, learning beings can change the invariances that govern their behavior. Therefore, we should not take the relationship between epistemic achievements and scientific acceptance as given. It might change in the future (or it might already be under change). It is possible that we either learn the wrong lessons from the past or change our interests in a way that changes the patterns of dependence between theory acceptance, values, and fruitfulness. For example, it is possible that we begin to pay attention on the moral status of a scientific group in the assessment of its results.[3] As Kuusi has insisted, learning beings can change their behavior and the rules that govern it.
Yet, we cannot overplay the extent to which learning beings change their behavior and rules. First, we saw above that it seems possible to find mid-range and structural invariances in human behavior. For example, it is clear that science has many actor-invariant features that govern the activities of individual scientists and that these features are not in a constant flux. For example, mathematical modelling has remained at the core of many sciences for a long time. Even if it is possible that we learn the implicit structure of scientific development and therefor change it (possibility discussed in a previous post), there is no evidence that such process is plausible. On the contrary, it seems that simplistic philosophical frameworks, such as falsificationism, have been recognized in science and cemented to the institutional discourses of science as definitions of what science “must always do”. Principles that were assumed to be invariant determinants of science have begun to dominate the normative structures of science rather than been abandoned. This means that recognizing (presumed) invariances does not, in fact, lead to a search for alternatives. Rather, it leads to the further strengthening of the invariances.
Secondly, science works at the intersection of human and natural world, and this seriously limits the learning processes of scientists. There are permanent invariances in the nature, like the properties of electrons, and these affect scientists. I have pointed out in a previous post that (at least sometimes) the behavior of scientists can only be explained by citing the features of the natural world, i.e. permanent invariances. The belief in the existence of electrons can only be understood if we know how the properties of electrons caused the outcome of the oil drop experiment. Historical human activities are embedded in wider order of invariances. The historical particulars of human activity cannot be understood without the wider order of invariances. This suggests that we can understand the history (and future!) of human activities by analyzing structural, mid-range, and low-level transient invariances against the background of known permanent invariances.
As a conclusion, I suggest that the notion of invariance connects scientific generalizations, historical explanation, and futures studies. Each of these fields require knowledge of invariances in order to achieve understanding. Moreover, knowledge of invariances in different fields enables to connect the results of the fields in our attempts to locate human activities in the causal processes from the past to the future.
Bob Dylan missed the fact that people might not be crazy nor the times strange. Things have changed but some things in human world remain invariant.
References
Bellon, Richard (2011). “Inspiration in the Harness of Daily Labor: Darwin, Botany, and the Triumph of Evolution, 1859–1868”. Isis: A Journal of the History of Science 102. 393-420.
Bolinska, Agnes & Martin, Joseph D. (2020). “Negotiating History: Contingency, Canonicity, and Case Studies.” Studies in History and Philosophy of Science 80:37–46.
Goertz, Gary (2012). “Descriptive-Causal Generalizations: “Empirical Laws” in the Social Sciences?” The Oxford Handbook of Philosophy of Social Science.
Kuhn, Thomas (1962). The Structure of Scientific Revolutions.
Kuukkanen, Jouni-Matti (2016). “Historicism and the failure of HPS”. Studies in History and Philosophy of Science.
Kuusi, Osmo (2019). ”Kuinka yhdentää tulevaisuuksientutkimusta, kognitiivista psykologiaa ja tekoälyn kehittämistä?”. Futura 2/19.
Kuusi, Osmo (1999). Expertise in The Future Use of Generic Technologies. Epistemic and Methodological Considerations Concerning Delphi Studies.
Virmajoki, Veli (2021). “Local Explanation in Historiography of Science”. European Journal of Philosophy of Science.
Woodward, James (2003). Making Things Happen.
[1] Notice, interestingly, that the existence of a one more planet would have been much stronger ontological commitment to Johannes Kepler, who proposed that the distance relationships between the six planets known at that time could be understood in terms of the five Platonic solids.
[2] Important evidence for Einstein’s theory consisted of the fact that the theory was able to calculate the precession even though the theory was formulated independently of the problem.
[3] Notice that it is a historical fact that many groups have been excluded from science because they were judged “inferior” in their cognitive abilities.